( Wiskunde - Getal en Ruimte 12de editie, VWO 2 , H7 Kwadratische vergelijkingen)
Diagnostische toets
(Opgave 2, 5, 7, 11, 13, 15)
Opgave 2.
→ "De horizontale lijn op hoogte – 3" is y = –3
→"De grafiek van y = – 2x² + 5 snijdt y = –3→ – 2x² + 5 = –3
– 2x² + 5 = –3+3 = +3–2x² + 8 = 0 ← Maak het rechterlid nul.–2x² –2∙(–4) = 0–2(x² – 4) = 0 ← Breng gemeenschappelijke factor –2 buiten haakjes.–2(x² – 2²) = 0 ← De regel a² – b² = (a – b)(a + b)–2(x – 2)(x + 2) = 0
x – 2 = 0 of x + 2 = 0 → x = 2 ∨ x = –2De y-coördinaat van punt A en B is –3, want y = –3.A(–2, –3) B(2, –3)
Opgave 5. Ontbind in zoveel mogelijk factoren.
a. 36x² – 24xy = 12∙3∙x∙x –12∙2∙x∙y
= 12x(3x –2y) ← Gemeenschappelijke factor 12x
b. 12x⁶ – 4x² = 4∙x²∙x⁴ – 4∙x² ← Gemeenschappelijke factor 4x²
= 4x²(x⁴ – 1) = 4x²((x²) ²– 1²)= 4x²(x² – 1²)(x² +1) ← De regel a² – b² = (a – b)(a + b)= 4x²(x – 1)(x + 1)(x² +1) ← De regel a² – b² = (a – b)(a + b)
c. 5x²y + xy – 2xy² = 5∙x∙x∙y + x∙y – 2∙x∙y∙y
= xy(5x + 1 – 2y) ← Gemeenschappelijke factor xy
d. 9x² – 121 = 3²x² – 11²
= (3x – 11)(3x +11) ← De regel a² – b² = (a – b)(a + b)
e. a³– a = a∙a² – a ← Gemeenschappelijke factor a
= a(a² – 1²)= a(a – 1)(a + 1) ← De regel a² – b² = (a – b)(a + b)
f. 60abc – 12a³c = 12∙5∙a∙b∙c – 12∙a∙a²∙c
= 12ac(5b – a²) ← Gemeenschappelijke factor 12ac
Opgave 7. Ontbind in zoveel mogelijk factoren.
a. x² + 10x + 21
+3
+7
= (x + 3)( x + 7) ← De Product-som-methode: 3 × 7 = 21 en 3 +7 = 10
b. x² + 10x – 24
+12
–2
= (x + 12)(x – 2) ← De Product-som-methode: 12 × –2 = –24 en 12 +(–2) = 10
c. 3x² – 4x = 3∙x∙x – 4∙x
= x(3x – 4) ← Gemeenschappelijke factor x
d. x² – 4x – 32
+4
–8
= (x + 4)(x – 8) ← De Product-som-methode: 4 × –8 = –32 en 4 +(–8) = –8
e. x² – 8x – 48
–12
+4
= (x – 12)(x + 4) ← De Product-som-methode: –12 × 4 = –48 en –12 + 4 = –8
f. x² + x – 56
–7
+8
= (x – 7)( x + 8) ← De Product-som-methode: –7 × 8 = –56 en –7 + 8 = 1
g. 2x³ – 128x = 2∙x∙x² – 2∙64∙x
= 2x(x² – 64) ← Gemeenschappelijke factor 2x= 2x(x² – 8²)= 2x(x – 8)(x + 8) ← De regel a² – b² = (a – b)(a + b)
h. 3x² – 6x – 9 = 3∙x² – 3∙2∙x – 3∙3
= 3(x² – 2x – 3) ← Gemeenschappelijke factor 3
–3
+1
= 3(x – 3)(x + 1) ← De Product-som-methode: –3 × 1 = –3 en –3 + 1 = –2
i. x³ + 9x² – 22x = x∙x² + 9∙x∙x – 22∙x
= x(x² + 9x – 22) ← Gemeenschappelijke factor x
–2
+11
= x(x – 2)(x +11) ← De Product-som-methode: –2 × 11 = –22 en –2 + 11 = 9
Opgave 11.
a.
→ "De coördinaten van de snijpunten met de x-as"→ De x-as ligt altijd op y = 0→ De grafiek van y = x²– 2x –3 snijdt de grafiek van y = 0.x²– 2x –3 = 0–3+1(x – 3)(x + 1) = 0 ← De Product-som-methode: –3× 1 = –3 en –3 + 1 = –2x – 3 = 0 of x + 1 = 0x = 3 ∨ x = –1
A(–1 , 0) en B(3, 0)
b.
→ "De coördinaten van de snijpunten met de x-as"→ De x-as ligt altijd op y = 0→ De grafiek van y = ⅓x² + x snijdt de grafiek van y = 0.⅓x² + x = 0× 3 = × 33×⅓x² + 3×x = 0×3 ← Vermenigvuldig beide zijden met 3x² + 3x = 0x∙x + 3∙x = 0x(x + 3) = 0 ← Gemeenschappelijke factor xx = 0 of x +3 = 0x = 0 ∨ x = –3
C(–3, 0) en O(0, 0)
Opgave 13.
→ De grafiek van y = x² – 5 snijdt de grafiek van y = 4x + 7."
x² – 5 = 4x + 7–4x – 7 = –4x – 7x² – 4x – 12 = 0 ← Maak het rechterlid nul.–6+2(x – 6)(x + 2) = 0 ←De Product-som-methode: –6× 2 = –12 en –6 + 2 = –4
x – 6 = 0 of x + 2 = 0x = 6 ∨ x = –2
→ Vind de y-coördinaten van de snijpunten door de x-coördinaten in de formule y = 4x + 7 in te vullen.① x = 6 → y = 4(6) + 7 = 24 + 7 = 31② x = –2 → y = 4(–2) + 7 = –8 + 7 = – 1De coördinaten van de snijpunten zijn (6, 31) en (–2, –1).
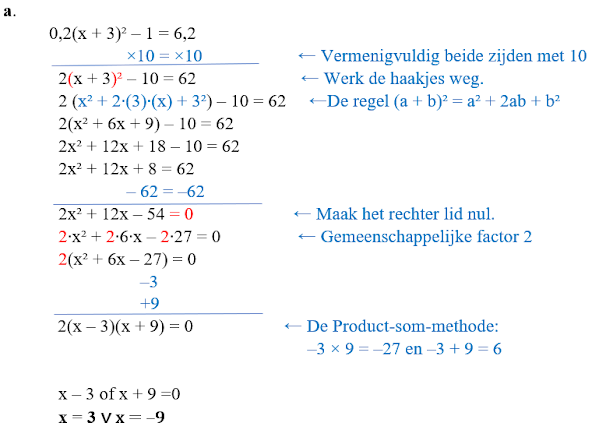
Opgave 15.
x = –14 ∨ x = 4 → x = 4, want x is een lengte. x is 4m.